Abstract from wikipedia
The effect occurs when two identical single-photon waves enter a 1:1 beam splitter, one in each input port. When the photons are identical, they will extinguish each other. If they become more distinguishable, the probability of detection will increase. In this way the interferometer can accurately measure bandwidth, path lengths and timing.
When a photon enters a beam splitter, there are two possibilities: it will either be reflected or transmitted. The relative probabilities of transmission and reflection are determined by the reflectivity of the beam splitter. Here, we assume a 1:1 beam splitter, in which a photon has equal probability of being reflected and transmitted.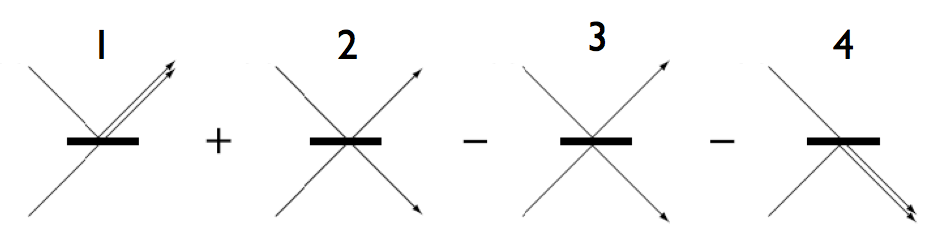
If the two photons are identical in their physical properties and indistinguishable, we cannot distinguish between the output states of possibilities 2 and 3.
Customarily the Hong–Ou–Mandel effect is observed using two photodetectors monitoring the output modes of the beam splitter. The coincidence rate of the detectors will drop to zero when the identical input photons overlap perfectly in time. This is called the Hong–Ou–Mandel dip, or HOM dip. The coincidence count reaches a minimum, indicated by the dotted line. The minimum drops to zero when the two photons are perfectly identical in all properties. When the two photons are perfectly distinguishable, the dip completely disappears.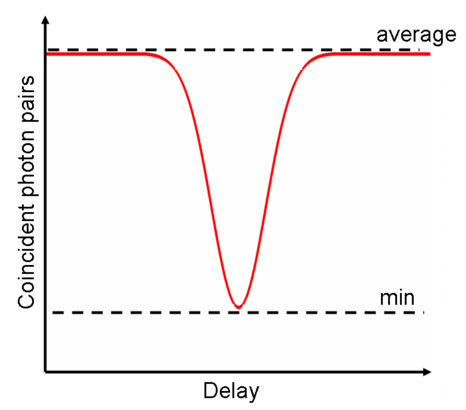
In reference 2, we can find
\begin{equation}
\begin{aligned}
\vert \psi_f \rangle&=\frac{1}{2\sqrt{2}}[(\alpha \gamma + \beta \delta)(\vert H\rangle_1 |H\rangle_2 + |V\rangle_1 |V\rangle_2 )\cdot i(|c\rangle_1 |c\rangle_2 + |d\rangle_1 |d\rangle_2)\\
&\phantom{=\frac{1}{2\sqrt{2}}}+(\alpha \gamma - \beta \delta)(\vert H\rangle_1 |H\rangle_2 - |V\rangle_1 |V\rangle_2 )\cdot i(|c\rangle_1 |c\rangle_2 + |d\rangle_1 |d\rangle_2)\\
&\phantom{=\frac{1}{2\sqrt{2}}}+(\alpha \delta + \beta \gamma)(\vert H\rangle_1 |V\rangle_2 + |V\rangle_1 |H\rangle_2 )\cdot i(|c\rangle_1 |c\rangle_2 + |d\rangle_1 |d\rangle_2)\\
&\phantom{=\frac{1}{2\sqrt{2}}}+(\alpha \delta - \beta \gamma)(\vert H\rangle_1 |V\rangle_2 - |H\rangle_1 |V\rangle_2 )\cdot (|d\rangle_1 |c\rangle_2 - |c\rangle_1 |d\rangle_2)]
\end{aligned}
\label{phif}
\end{equation}
The probability of 2 and 3 changes from 50% to \(\frac{1}{8}|\alpha\delta-\beta\gamma|^2\)
Reference
- https://en.wikipedia.org/wiki/Hong%E2%80%93Ou%E2%80%93Mandel_effect
- Quantum Teleportation and Multi-photon Entanglement, Jian-Wei Pan